
views
Converting An Improper Fraction
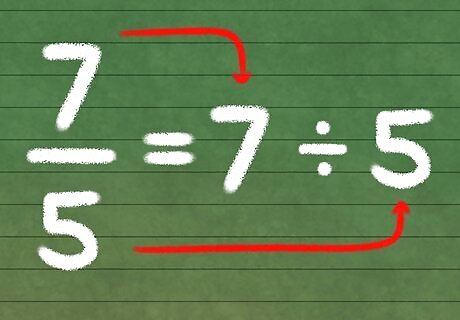
Divide the numerator by the denominator. Start by writing your improper fraction. Then, divide the numerator by the denominator — in other words, just do the division problem that the fraction is already set up for. Don't forget to include the remainder. Let's follow along with an example. Let's say that we need to turn the fraction 7/5 into a mixed number. We'll start by dividing 7 by 5, like this: 7/5 → 7 ÷ 5 = 1 R2
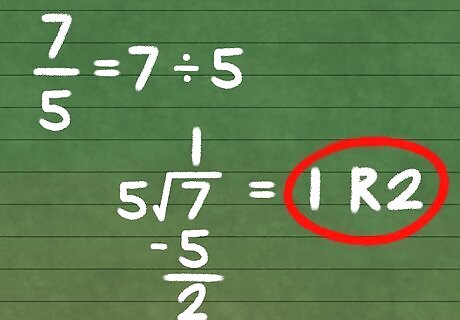
Write the whole number answer. The whole number part of your mixed number (the big number to the left of your fraction) is the whole number answer of your division problem. In other words, just write the answer of the division problem without the remainder. In our example, since our answer is 1 R2, we would leave off the remainder and just write 1.
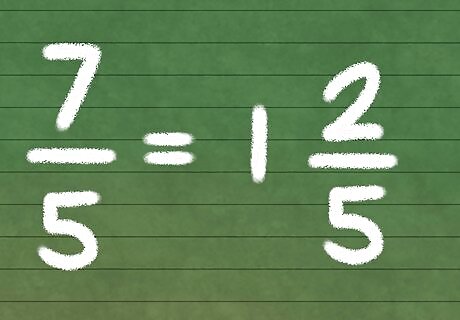
Make a fraction from the remainder and the original denominator. Now, we need to find the fraction part of the mixed number. Put the remainder from your division problem in the numerator and use the same denominator from your original improper fraction. Put this fraction next to your whole number and you have your mixed number! In our example, our remainder is 2. Putting this over our original denominator (5), we get 2/5. We put this next to our whole number answer (1) to get our final mixed number, like this: 1 2/5.
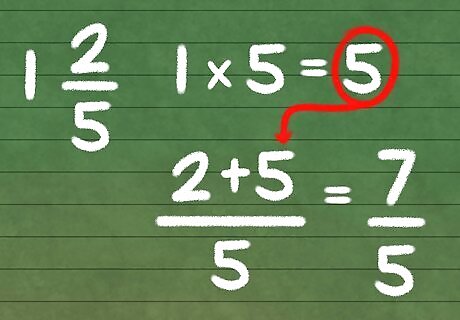
To get back to an improper fractions, add the whole number to the numerator. Mixed numbers look good on paper and are easy to read, but they're not always the best choice. For example, if we're multiplying a fraction and a mixed number, our work will be a lot easier if we convert the mixed number back into an improper fraction. To do this, just multiply the whole number by the denominator and add it to the numerator. If we wanted to convert our example answer (1 2/5) back to an improper fraction, we would do it like this: 1 × 5 = 5 → (2 + 5)/5 = 7/5
Solving Sample Problems
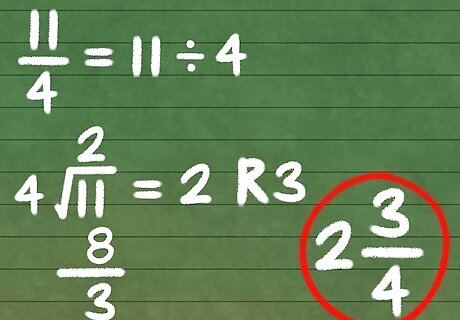
Convert 11/4 to a mixed number. This problem is easy — just solve exactly as above. See below for a step-by-step solution. 11/4 — to start, we need to divide the numerator by the denominator. 11 ÷ 4 = 2 R 3 — now, we need to make a fraction from the remainder and our original denominator. 11/4 = 2 3/4
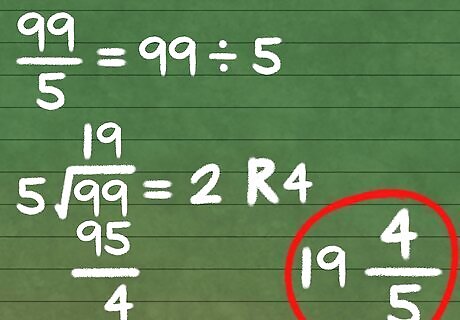
Convert 99/5 to a mixed number. We're dealing with a really big numerator here, but don't be intimidated — the process is exactly the same! See below: 99/5 — how many times does 5 go into 99? Since 5 goes into 100 exactly 20 times, it's safe to say that 5 goes into 99 19 times. 99 ÷ 5 = 19 R 4 — now, we just put the mixed number together like before. 99/5 = 19 4/5
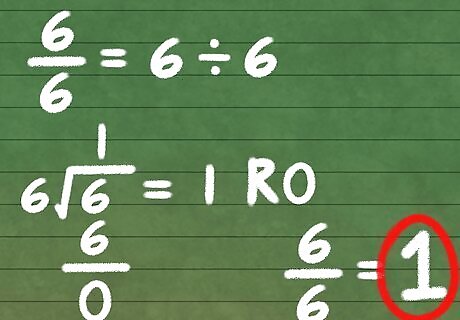
Convert 6/6 to a mixed number. Up until now, we've only dealt with improper fractions where the numerator is bigger than the denominator. But what happens when they're the same number? See below to find out. 6/6 — six goes into six one time with no remainder, obviously. 6 ÷ 6 = 1 R0. Since a fraction with 0 in the numerator is always equal to zero, we don't need to put a fraction next to our whole number. 6/6 = 1
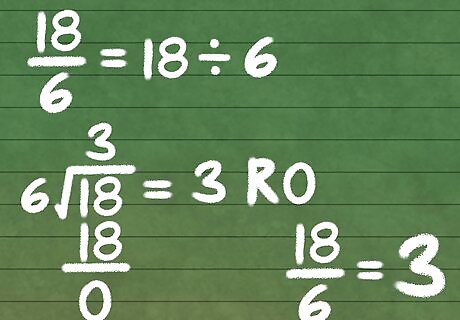
Convert 18/6 to a mixed number. If the numerator is a multiple of the denominator, you don't have to bother with the remainder — just do the division problem to get your answer. See below. 18/6 — since we know that 18 is just 6 × 3, we know we'll have a remainder of 0, so we don't need to worry about the fraction part of our mixed number. 18/6 = 3
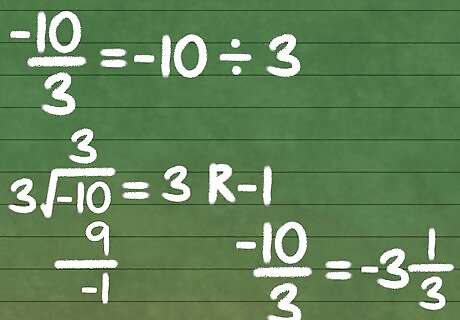
Convert -10/3 to a mixed number. Negatives work exactly the same way as positive numbers do. See below: -10/3 -10 ÷ 3 = -3 R1 -10/3 = -3 1/3


















Comments
0 comment